IB Physics Galileo's Pendulum
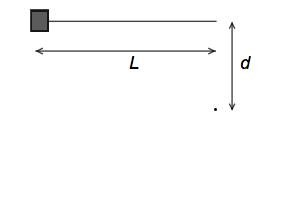
/A tutorial sheet on Galileo’s pendulum, a pendulum where the string strikes a peg as the pendulum swings, is given below. The length of the string is L and the peg is a distance d below the point of support of the string.
- In the diagram the mass is released from rest on the end of a light inextensible string with the string initially horizontal. Determine the speed of the mass at the lowest point in its swing.
- In question 1, d = 0.5L. Find the maximum height reached by the mass above the peg after the string strikes the peg.
- In question 1 show that d must be at least 0.6L if the mass is to swing completely around the peg.
- The mass is placed on a string of length 1.0 m and released with the string making an angle of 60° with the vertical. If d = 0.7 m, find the angle made by the string with the horizontal when the mass reaches its highest point. (41.8°)
- If d = 2L/3, find the least angle to the vertical at which the string can be released if the mass is to swing completely around the peg. (80.41°)
- The string is replaced by an elastic spring of force constant k. The mass m is released from rest with the spring horizontal and unextended. Is the speed of the mass at the bottom of the swing greater than, equal to or less than, the speed of the mass when the string is inelastic?